Update

xjtuchenchao · Apr 22, 2021 · 42eb277 · 42eb277
1 parent b4fefff
commit 42eb277
Show file tree

Hide file tree

Showing 3 changed files with 494 additions and 2 deletions.
diff --git a/README.md b/README.md
@@ -251,8 +251,8 @@
 4. [回溯算法：求组合总和！](./problems/0216.组合总和III.md)
 5. [回溯算法：电话号码的字母组合](./problems/0017.电话号码的字母组合.md)
 6. [本周小结！（回溯算法系列一）](./problems/周总结/20201030回溯周末总结.md)
-7. [回溯算法：求组合总和（二）](https://mp.weixin.qq.com/s/FLg8G6EjVcxBjwCbzpACPw)
-8. [回溯算法：求组合总和（三）](https://mp.weixin.qq.com/s/_1zPYk70NvHsdY8UWVGXmQ)
+7. [回溯算法：求组合总和（二）](./problems/0039.组合总和.md)
+8. [回溯算法：求组合总和（三）](./problems/0040.组合总和II.md)
 9. [回溯算法：分割回文串](https://mp.weixin.qq.com/s/Pb1epUTbU8fHIht-g_MS5Q)
 10. [回溯算法：复原IP地址](https://mp.weixin.qq.com/s/v--VmA8tp9vs4bXCqHhBuA)
 11. [回溯算法：求子集问题！](https://mp.weixin.qq.com/s/NNRzX-vJ_pjK4qxohd_LtA)

diff --git a/problems/0039.组合总和.md b/problems/0039.组合总和.md
@@ -0,0 +1,236 @@
+
+<p align="center">
+  <a href="https://mp.weixin.qq.com/s/QVF6upVMSbgvZy8lHZS3CQ"><img src="https://img.shields.io/badge/知识星球-代码随想录-blue" alt=""></a>
+  <a href="https://mp.weixin.qq.com/s/b66DFkOp8OOxdZC_xLZxfw"><img src="https://img.shields.io/badge/刷题-微信群-green" alt=""></a>
+  <a href="https://img-blog.csdnimg.cn/20201210231711160.png"><img src="https://img.shields.io/badge/公众号-代码随想录-brightgreen" alt=""></a>
+  <a href="https://space.bilibili.com/525438321"><img src="https://img.shields.io/badge/B站-代码随想录-orange" alt=""></a>
+</p>
+
+## 39. 组合总和
+
+题目链接：https://leetcode-cn.com/problems/combination-sum/
+
+给定一个无重复元素的数组 candidates 和一个目标数 target ，找出 candidates 中所有可以使数字和为 target 的组合。
+
+candidates 中的数字可以无限制重复被选取。
+
+说明：
+
+* 所有数字（包括 target）都是正整数。
+* 解集不能包含重复的组合。 
+
+示例 1：
+输入：candidates = [2,3,6,7], target = 7,
+所求解集为：
+[
+  [7],
+  [2,2,3]
+]
+
+示例 2：
+输入：candidates = [2,3,5], target = 8,
+所求解集为：
+[
+  [2,2,2,2],
+  [2,3,3],
+  [3,5]
+]
+
+## 思路
+
+[B站视频讲解-组合总和](https://www.bilibili.com/video/BV1KT4y1M7HJ)
+
+
+题目中的**无限制重复被选取，吓得我赶紧想想 出现0 可咋办**，然后看到下面提示：1 <= candidates[i] <= 200，我就放心了。
+
+本题和[回溯算法：求组合问题！](https://mp.weixin.qq.com/s/OnBjbLzuipWz_u4QfmgcqQ)，[回溯算法：求组合总和！](https://mp.weixin.qq.com/s/HX7WW6ixbFZJASkRnCTC3w)和区别是：本题没有数量要求，可以无限重复，但是有总和的限制，所以间接的也是有个数的限制。
+
+本题搜索的过程抽象成树形结构如下：
+
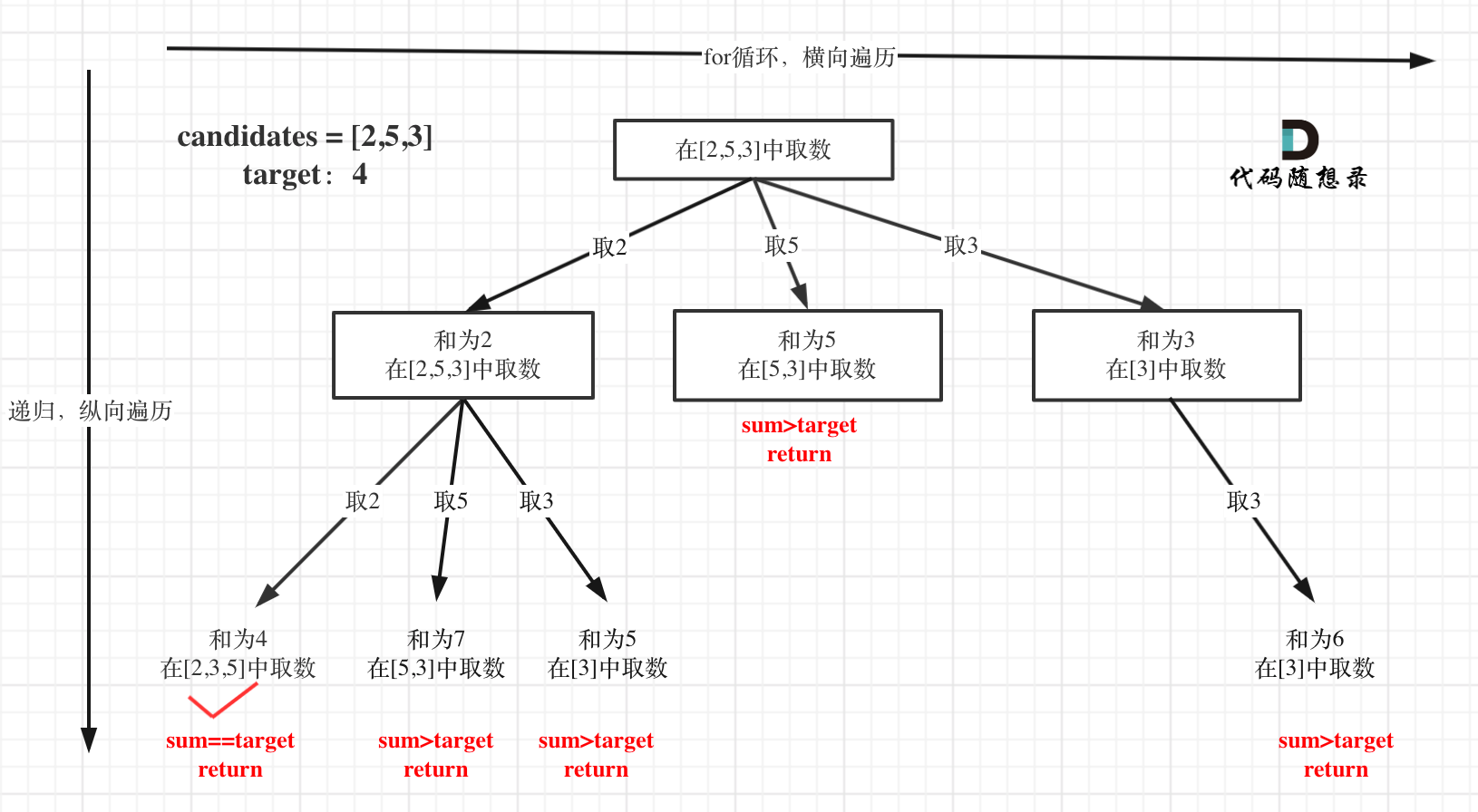+![39.组合总和](https://img-blog.csdnimg.cn/20201223170730367.png)
+注意图中叶子节点的返回条件，因为本题没有组合数量要求，仅仅是总和的限制，所以递归没有层数的限制，只要选取的元素总和超过target，就返回！
+
+而在[回溯算法：求组合问题！](https://mp.weixin.qq.com/s/OnBjbLzuipWz_u4QfmgcqQ)和[回溯算法：求组合总和！](https://mp.weixin.qq.com/s/HX7WW6ixbFZJASkRnCTC3w) 中都可以知道要递归K层，因为要取k个元素的组合。
+
+## 回溯三部曲
+
+* 递归函数参数
+
+这里依然是定义两个全局变量，二维数组result存放结果集，数组path存放符合条件的结果。（这两个变量可以作为函数参数传入）
+
+首先是题目中给出的参数，集合candidates, 和目标值target。
+
+此外我还定义了int型的sum变量来统计单一结果path里的总和，其实这个sum也可以不用，用target做相应的减法就可以了，最后如何target==0就说明找到符合的结果了，但为了代码逻辑清晰，我依然用了sum。
+
+**本题还需要startIndex来控制for循环的起始位置，对于组合问题，什么时候需要startIndex呢？**
+
+我举过例子，如果是一个集合来求组合的话，就需要startIndex，例如：[回溯算法：求组合问题！](https://mp.weixin.qq.com/s/OnBjbLzuipWz_u4QfmgcqQ)，[回溯算法：求组合总和！](https://mp.weixin.qq.com/s/HX7WW6ixbFZJASkRnCTC3w)。
+
+如果是多个集合取组合，各个集合之间相互不影响，那么就不用startIndex，例如：[回溯算法：电话号码的字母组合](https://mp.weixin.qq.com/s/e2ua2cmkE_vpYjM3j6HY0A)
+
+**注意以上我只是说求组合的情况，如果是排列问题，又是另一套分析的套路，后面我再讲解排列的时候就重点介绍**。
+
+代码如下：
+
+```C++
+vector<vector<int>> result;
+vector<int> path;
+void backtracking(vector<int>& candidates, int target, int sum, int startIndex)
+```
+
+* 递归终止条件
+
+在如下树形结构中：
+
+![39.组合总和](https://img-blog.csdnimg.cn/20201223170730367.png)
+
+从叶子节点可以清晰看到，终止只有两种情况，sum大于target和sum等于target。
+
+sum等于target的时候，需要收集结果，代码如下：
+
+```C++
+if (sum > target) {
+    return;
+}
+if (sum == target) {
+    result.push_back(path);
+    return;
+}
+```
+
+* 单层搜索的逻辑
+
+单层for循环依然是从startIndex开始，搜索candidates集合。
+
+**注意本题和[回溯算法：求组合问题！](https://mp.weixin.qq.com/s/OnBjbLzuipWz_u4QfmgcqQ)、[回溯算法：求组合总和！](https://mp.weixin.qq.com/s/HX7WW6ixbFZJASkRnCTC3w)的一个区别是：本题元素为可重复选取的**。
+
+如何重复选取呢，看代码，注释部分：
+
+```C++
+for (int i = startIndex; i < candidates.size(); i++) {
+    sum += candidates[i];
+    path.push_back(candidates[i]);
+    backtracking(candidates, target, sum, i); // 关键点:不用i+1了，表示可以重复读取当前的数
+    sum -= candidates[i];   // 回溯
+    path.pop_back();        // 回溯
+}
+```
+
+按照[关于回溯算法，你该了解这些！](https://mp.weixin.qq.com/s/gjSgJbNbd1eAA5WkA-HeWw)中给出的模板，不难写出如下C++完整代码：
+
+```C++
+// 版本一
+class Solution {
+private:
+    vector<vector<int>> result;
+    vector<int> path;
+    void backtracking(vector<int>& candidates, int target, int sum, int startIndex) {
+        if (sum > target) {
+            return;
+        }
+        if (sum == target) {
+            result.push_back(path);
+            return;
+        }
+
+        for (int i = startIndex; i < candidates.size(); i++) {
+            sum += candidates[i];
+            path.push_back(candidates[i]);
+            backtracking(candidates, target, sum, i); // 不用i+1了，表示可以重复读取当前的数
+            sum -= candidates[i];
+            path.pop_back();
+        }
+    }
+public:
+    vector<vector<int>> combinationSum(vector<int>& candidates, int target) {
+        result.clear();
+        path.clear();
+        backtracking(candidates, target, 0, 0);
+        return result;
+    }
+};
+```
+
+## 剪枝优化
+
+在这个树形结构中：
+
+![39.组合总和](https://img-blog.csdnimg.cn/20201223170730367.png)
+
+以及上面的版本一的代码大家可以看到，对于sum已经大于target的情况，其实是依然进入了下一层递归，只是下一层递归结束判断的时候，会判断sum > target的话就返回。
+
+其实如果已经知道下一层的sum会大于target，就没有必要进入下一层递归了。
+
+那么可以在for循环的搜索范围上做做文章了。
+
+**对总集合排序之后，如果下一层的sum（就是本层的 sum + candidates[i]）已经大于target，就可以结束本轮for循环的遍历**。
+
+如图：
+
+
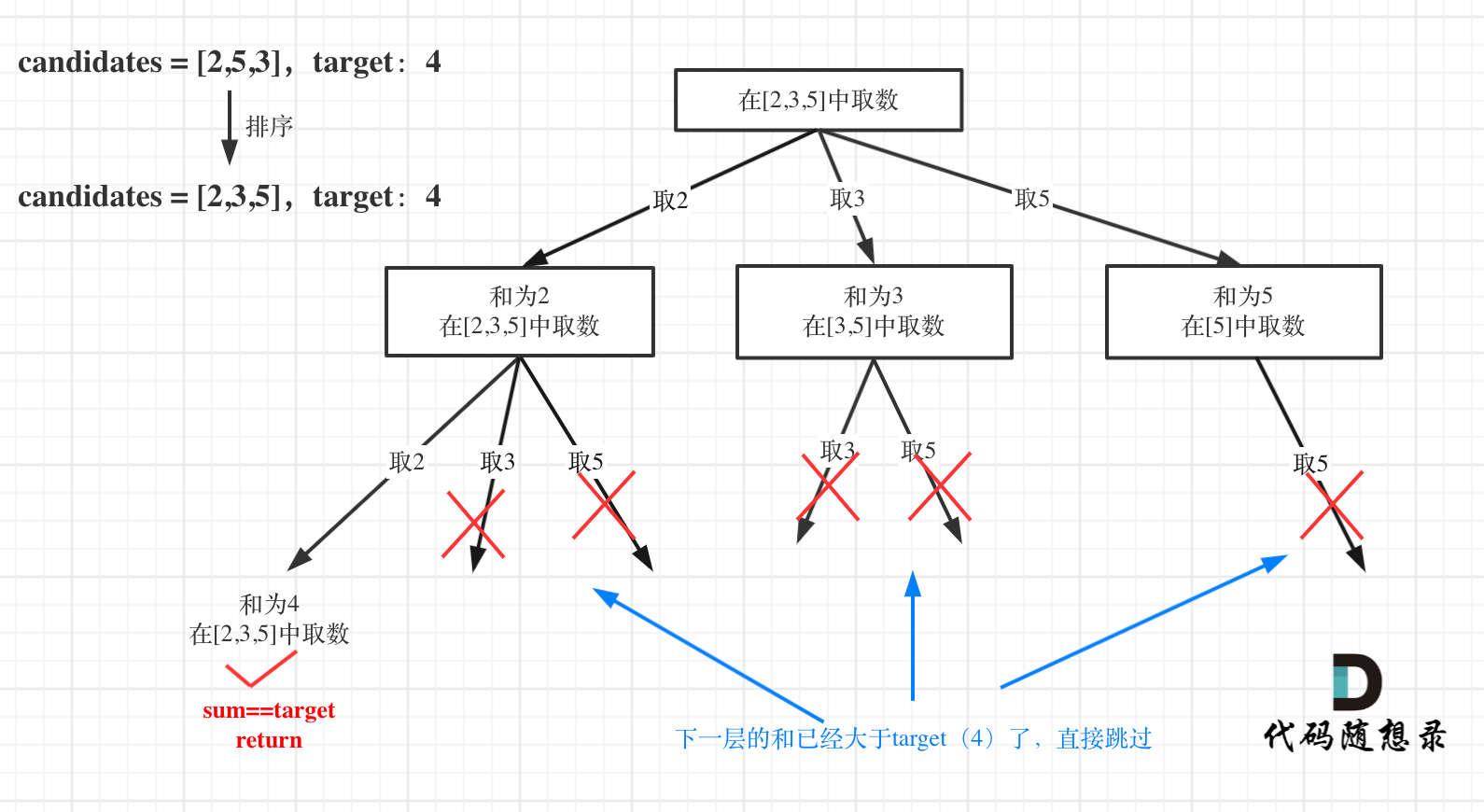+![39.组合总和1](https://img-blog.csdnimg.cn/20201223170809182.png)
+
+for循环剪枝代码如下：
+
+```
+for (int i = startIndex; i < candidates.size() && sum + candidates[i] <= target; i++)
+```
+
+整体代码如下：（注意注释的部分）
+
+```C++
+class Solution {
+private:
+    vector<vector<int>> result;
+    vector<int> path;
+    void backtracking(vector<int>& candidates, int target, int sum, int startIndex) {
+        if (sum == target) {
+            result.push_back(path);
+            return;
+        }
+
+        // 如果 sum + candidates[i] > target 就终止遍历
+        for (int i = startIndex; i < candidates.size() && sum + candidates[i] <= target; i++) {
+            sum += candidates[i];
+            path.push_back(candidates[i]);
+            backtracking(candidates, target, sum, i);
+            sum -= candidates[i];
+            path.pop_back();
+
+        }
+    }
+public:
+    vector<vector<int>> combinationSum(vector<int>& candidates, int target) {
+        result.clear();
+        path.clear();
+        sort(candidates.begin(), candidates.end()); // 需要排序
+        backtracking(candidates, target, 0, 0);
+        return result;
+    }
+};
+```
+
+## 总结
+
+本题和我们之前讲过的[回溯算法：求组合问题！](https://mp.weixin.qq.com/s/OnBjbLzuipWz_u4QfmgcqQ)、[回溯算法：求组合总和！](https://mp.weixin.qq.com/s/HX7WW6ixbFZJASkRnCTC3w)有两点不同：
+
+* 组合没有数量要求
+* 元素可无限重复选取
+
+针对这两个问题，我都做了详细的分析。
+
+并且给出了对于组合问题，什么时候用startIndex，什么时候不用，并用[回溯算法：电话号码的字母组合](https://mp.weixin.qq.com/s/e2ua2cmkE_vpYjM3j6HY0A)做了对比。
+
+最后还给出了本题的剪枝优化，这个优化如果是初学者的话并不容易想到。
+
+**在求和问题中，排序之后加剪枝是常见的套路！**
+
+可以看出我写的文章都会大量引用之前的文章，就是要不断作对比，分析其差异，然后给出代码解决的方法，这样才能彻底理解题目的本质与难点。
+
+------------------------
+
+* 微信：[程序员Carl](https://mp.weixin.qq.com/s/b66DFkOp8OOxdZC_xLZxfw)
+* B站：[代码随想录](https://space.bilibili.com/525438321)
+* 知识星球：[代码随想录](https://mp.weixin.qq.com/s/QVF6upVMSbgvZy8lHZS3CQ)
+
+![](https://img-blog.csdnimg.cn/20210416110157800.png)